Lengthleaper Hex Chess
The idea for this variant came from wishing to demonstrate easy definition of hex-prism leapers. This is because Armies of Faith 2 would use such a board to bind certain pieces to particular levels and accommodate some specifically hex pieces. While planning that variant I decided to first produce a smaller, same-armies, and unthemed hex-prism variant with non-square pieces rather than add a new way to define pieces on top of the other complexities of a themed one. Hopefully this also stands up as a game in its own right.Deciding against using AOF2 pieces this early on, I wondered what pieces would best illustrate the general principle of describing hex-prism pieces. I had noticed that hex-prism pieces sharing a leap length proliferate compared to cubic ones. The first 8 values of Square Of Leap Length (SOLL) with multiple cubic leapers are 9, 17, 18, 25, 26, 27, 33, and 34 - see Man and Beast 05: Punning by Numbers - whereas the first 8 with multiple hex-prism leapers are 4, 7, 8, 12, 13, 16, 17, and 19 - see Man and Beast 14: Oddly Oblique. I therefore decided on leap-length-based compound pieces with leaps up to and including the square root of 17 (r17 in the notation to be used here), and the latter article describes many of their components. These pieces also serve to illustrate how 2d hex pieces on a hex-prism board are bound to particular hex boards.
Every hex diagonal is at right angles to both a hex orthogonal and the interhex orthogonal. This means that each combination of an otrhofonbal coordinate and a hex-diagonal coordinate represents both a purely hex piece and a hex-prism pece which is a 90° rotation of the hex piece. These are highlighted in the descriptions of the compuond pieces.
An AOF2 feature that I did use here was the orientation. Honeycomb Chess has a FIDE-like orientation using hex boards as ranks, but that has problems as it gives Pawns 6 forward diagonals to only one forward orthogonal. I had however already covered the transition to the orientation used here, in 3-player Honeycomb Chess. That has a better Pawn move ratio. At least four levels are required as two pieces have components moving three levels, so I chose that minimal number. I decided on 2 players for simplicity's sake even though this orientation (unlike hex boards as ranks) allows 3, and chose the remaining dimensions accordingly.
To devise a suitable way to define hex-prism leapers I exploited the fact that, on the simple hex board in 2d, multiple-leaper SOLLs are conversely very rare. The first is 49, which has both a radial and an oblique leaper. This allows most leapers in that geometry to be defined uniquely by leap length - just like on a 1d board but with non-integer leap-lengths as well. This allows each hex-prism leaper to be defined by just two coordinates. One is the number of levels moved and must be an integer; the other is the distance between columns, and need not be an integer but must be the square root of one. If both coordinates are integers either can be the number of levels moved, for example on the board below a Knight would be able to move 2 levels and 1 column or 1 level and 2 columns, just like ranks and files in FIDE Chess. If zero is the only integer the piece is bound to a single hex board.
I have now devised a subvariant whose radial pieces are also more hex-specific. As it uses the same board I have incorporated it on this page.
The page name includes hex just in case I ever try a Lengthleaper Cubic Chess - although the need to resort to longer leaps would, I suspect, detract from the game.
Setup
The board has 4 levels by 34 columns. Levels are lettered a (highest - Bishops), b (second highest, King/Queen), c (second lowest - Fourleapers), d (lowest - Rooks). Columns are numbered as follows:
86 85 84
76 75 74 73
66 65 64 63 62
56 55 54 53 52 51
45 44 43 42 41
34 33 32 31
23 22 21
12 11
Columns dividing by 3 exactly are yellow on levels a/c and purple on levels b/d.
Columns dividing by 3 with remainder 1 are blue on levels a/c and orange on levels b/d.
Columns dividing by 3 with remainder 2 are red on levels a/c and green on levels b/d.
Individual cells have the level letter interposed between the column digits. Ranks are series of columns with the same first digit.
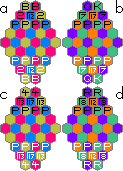
This is the subvariant replacing Rooks and Bishops with hex-specific radial pieces. As the directions of these are based on applying the cubic-board angles to a hex-prism board I have used notional move diagrams to represent them. Devising names for them was quite a task as I hoped to give short/long pairs with an orthogonal component a male/female pair of names. As I had a certain species of bird in mind for reasons that would soon become clear, and it had distinctive names for the two sexes, I developed this theme with some bird names previously unused for pieces. This ties in rather well with the Finch in my 2d hex variant Anglojewish Chess.

Pieces
In the older subvariant each player has a King, a Queen, and 16 Pawns, and 2 of everything else; in the newer one each player has 1 each of all radial pieces, no Twoleapers (part of its move being represented elsewhere), and 2 of everything else. Note that "level" in the descriptions below corresponds to "rank" in a hex-ranked orientation.FIDE pieces are less changed from 2d than they would be on a cubic board, but with the significance of ranks for symmetric pieces switched to levels.
The KING moves one step in any of the 8 orthogonal and 12 root-2 diagonal directions. It must be kept out of Check.
The QUEEN moves as a Rook or a Bishop - see below.
The ROOK moves any distance through empty intermediate cells along Hex orthogonals within levels, cycling through the level's three colours, or along columns between levels, alternating between the column's two opposite colours.
The BISHOP moves any distance through empty intermediate cells along square diagonals, always changing level and column at the same rate, as it does file and rank in 2d, and cycling through all six colours in the obvious spectral order. In this geometry Bishops are unbound, but have no same-level move.
The PAWN moves one column toward the enemy camp, on the same level when not capturing and up/down one level when capturing. This gives 2 noncapturing and 4 capturing directions. Note that unlike a Raumschach (or AOF1) Pawn it lacks the fifth capturing direction between the two noncapturing ones, as this board has no same-level root-2 diagonal.
Length leapers are pieces leaping a fixed distance regardless of direction. Many with only two components have alternative names which can be extrapolated to part-symmetric and mixed-range compounds. Their names are explained in my piece articles Man and Beast 18: Complex Pieces with Simple Ratios and Man and Beast 19: The Vice Squad
| piece | components | resulting bnding | coordinates in orthogonal/hex-diagonal steps | notes |
|---|---|---|---|---|
| TWOLEAPER | 2:0 Dabbaba, moving 2 steps along any orthogonal and bound to a quarter of alternate levels r3:1 Rumbaba, moving 1 step along any hybrid diagonal and bound to a third of every level | unbound as the bindings are independent. | 1/1 | |
| ROOTSEVENLEAPER or HORSINESS | r7:0 Sennight, bound to all of a single level 2:r3 Foal, bound to a third of alternate levels | bound to the whole of alternate levels | 2/1 | Each player has one odd-levels and one even-levels Horsiness. |
| ROOTEIGHTLEAPER or HEADOFHERD | 2:2 Elephant (Alfil), bound to a quarter of alternate levels r7:1 Heffalump, unbound | unbound as Heffalump is | ||
| ROOTTWELVELEAPER or PERMIT | r12:0 Eunuch, bound to a meagre 12th of a single level 3:r3 Prefect, bound to a third of every 3rd level | inherits the Prefect's binding as Eunuch's is a subset | 3/3 | placed on the top and bottom levels as there are only 4 levels |
| ROOTTHIRTEENLEAPER | 3:2 Zebra, unbound on this board r13:0 Aurochs (see also Trios Hex Chess), bound to all of a single level r12:1 Baker, bound to a 12th of every level | unbound as Zebra is | 1/2 (not Zebra) | |
| FOURLEAPER | 4:0 Cobbler, moving 4 steps along any orthogonal and bound to a 16th of every 4th level r12:2 Drummer (see Man and Beast 17: Hex Heavies), moving 2 steps along any hybrid diagonal and bound to a 12th of alternate levels 3:r7 Sabbaba, bound to all of every 3rd level | unbound but unwieldy | 2/2 (not Sabbaba) | |
| ROOTSEVENTEENLEAPER or TOPHAT | 4:1 Giraffe, unbound on a large enough hex-prism or cubic board but bound to a 16th of every level if there are 4 or fewer r13:2 Fencer, bound to all of alternate levels | unbound even on this board | N/A |
Hex-specific radial pieces are all bound to the odd ranks. Other orientations would bind them to alternate levels (hex-ranked) or alternate files (Glinski levels).
The MOORCOCK (small +) moves one step straight forward/backward/sideways in a 2d hex board, and also straight up/down on a hex-prism one. It differs from a rectlinear-board Wazir (with which it shares the number of directions) in that one pair of directions is a hex diagonal. In this variant with its Wellisch levels that pair is forward/backward. In other orientations it would be up/down (hex-ranked) or sideways (Glinski levels). Overall its move is a subset of that of the hex or hex-prism Duke (Wazir+Viceroy), but it is with the cubic Duke that it shares the inability to lose the move. It also has parallels with the Ferz on a square board in that it is bound to half the board, but switches between halves of that half. All these properties apply in both 2d and 3d. The name means a male moorfowl, a relative of the grouse.
The MOORHEN (large +) makes any number of Moorcock steps in the same direction, of which only the last step can be capturing. Overall its move is a subset of that of the hex or hex-prism Duchess (Rook+Unicorn). The name refers to various birds of which the female moorfowl is most relevant here.
The GROUSE (small x) moves to any of the 8 cells immediately above or below any on-the-level Moorcock destination. In other orientations the directions are rotated accordingly. Overall its move is a subset of that of the Landlord (Ferz+Rumbaba), and like that piece it is specific to 3d and can never lose the move as it always switches from an odd to an even level or vice versa.
The PHEASANT (large x) makes any number of Grouse steps in the same direction, of which only the last can be capturing. Overall its move is a subset of that of the Pontiff (Bishop+Dicorn), and like that piece it is specific to 3d.
The PEACOCK (small *) is the compound of Moorcock and Grouse. Overall its move is a subset of that of the Worldgeneral (Wazir+Ferz+Viceroy+Rumbaba), and like that piece it is specific to 3d and can triangulate in 2 Wazir and one Ferz steps all within rank or a combination of either such step with a Viceroy step forward and Rumbaba one backward or vice versa.
The PEAHEN (large *) is the compound of Moorhen and Pheasant. Overall its move is a subset of that of the Worldrider (Rook+Bishop+Unicorn+Dicorn), and like that piece it is specific to 3d.
Rules
Pawns have an optional double-step noncapturing first move. This is appropriate as the Pawns have to go as far as FIDE Pawns to reach their promotion rank (see below). When making such a move a Pawn can be captured En Passant by an enemy Pawn (but by no other piece) as if it had made only the single-step move.There is no Castling.
A Pawn reaching the enemy King or Queen column must be promoted to one of the variant's length leapers. It cannot be promoted to a Roottwelve except on a Bishop or Rook cell (or their equivalents in the newer subvariant).
Check, Checkmate, and Stalemate are as standard.
Notes
Pieces in the older subvariant can be represented by one large and two small sets, with the large King/Queen/Rooks/Bishops and small Pawns representing themselves. Of the length leapers those expected to be most popular as promotees should be represented by large Pawns and those expected to be next most popular by small Bishops/Knights. Promotions to a piece of which there are only two representations can be done with a small Rook upturned, surmounted by a Pawn, or both.The 36 pieces aside allow a more economical - and less space-hungry in the vertical dimension - alternative of representing each army with a complete Shogi set minus Goldgenerals. For example, the King and Queen could be represented by promoted and unpromoted Bishops, Rooks by Shogi Rooks, Bishops by Shogi Kings, the length leapers expected to be most popular as promotees by promoted and Pawns by unpromoted Points, and the six other kinds of length leaper by the remaining three Shogi piece types promoted and unpromoted. Goldgenerals can be brought in alone to duplicate a second promotee type (not necessarily the same for both armies) or surmounting a Point to duplicate a third.
These physical sets can just about work for the newer subvariant as well, as FIDE sets have 7 representations if Rooks are counted both ways up and Shogi pieces have 14 markings between them.
As this is a 2-player one with an odd number of ranks each type of hex-specific radial piece has the same directions and binding for both players. An even number of ranks would give the same directions but opposite bindings. In a 3-player game their directions would differ according to each player's sense of, for example, "straight forward", and their bindings be independent. Players would have no Grouse/Pheasant directions in common, and only the vertical directions of the rest. For each piece the three players' moves would add up to the full symmetric compound mentioned under its entry in the Pieces section above.
Partidge is not used as the name of a hex-specific radial piece as is already has a meaning as a forward-only Hopping piece, an extrapolation from Cartridge.
 This 'user submitted' page is a collaboration between the posting user and the Chess Variant Pages. Registered contributors to the Chess Variant Pages have the ability to post their own works, subject to review and editing by the Chess Variant Pages Editorial Staff.
This 'user submitted' page is a collaboration between the posting user and the Chess Variant Pages. Registered contributors to the Chess Variant Pages have the ability to post their own works, subject to review and editing by the Chess Variant Pages Editorial Staff.
By Charles Gilman.
Web page created: 2007-07-07. Web page last updated: 2013-12-08
